State whether abc and aed are congruent justify your answer – State whether ABC and AED are congruent: a question that delves into the fascinating realm of geometry, where shapes and their properties take center stage. This exploration unravels the intricacies of congruence, providing a clear understanding of its significance and the methods used to determine it.
Congruence, a fundamental concept in geometry, establishes the equality of shapes and their corresponding parts. Understanding its conditions and implications is crucial for comprehending geometric relationships and solving complex problems. This analysis embarks on a journey to determine the congruence of shapes ABC and AED, employing logical reasoning and geometric principles to uncover the truth.
Understanding Congruence
In geometry, congruence refers to the equality of shapes in terms of their size and shape. Two shapes are congruent if they have the same dimensions and angles, regardless of their orientation or position.
Congruent shapes possess several key properties:
- Equal side lengths (for line segments or sides of polygons)
- Equal angles (for angles or interior angles of polygons)
- Identical overall shape (same number of sides, vertices, etc.)
For two shapes to be congruent, they must meet certain conditions:
- SSS (Side-Side-Side): If all three sides of one shape are equal to the corresponding sides of another shape, then the shapes are congruent.
- SAS (Side-Angle-Side): If two sides and the included angle of one shape are equal to the corresponding sides and included angle of another shape, then the shapes are congruent.
- ASA (Angle-Side-Angle): If two angles and the included side of one shape are equal to the corresponding angles and included side of another shape, then the shapes are congruent.
- AAS (Angle-Angle-Side): If two angles and a non-included side of one shape are equal to the corresponding angles and non-included side of another shape, then the shapes are congruent.
Identifying Congruent Shapes: State Whether Abc And Aed Are Congruent Justify Your Answer
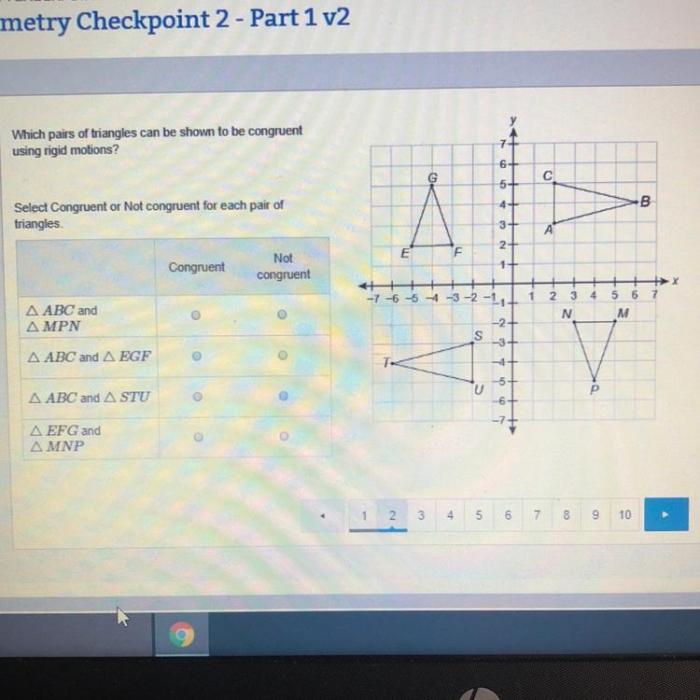
To determine whether two shapes are congruent, follow these steps:
- Compare the side lengths and angles of the shapes.
- Apply the congruence conditions (SSS, SAS, ASA, AAS) to see if they match.
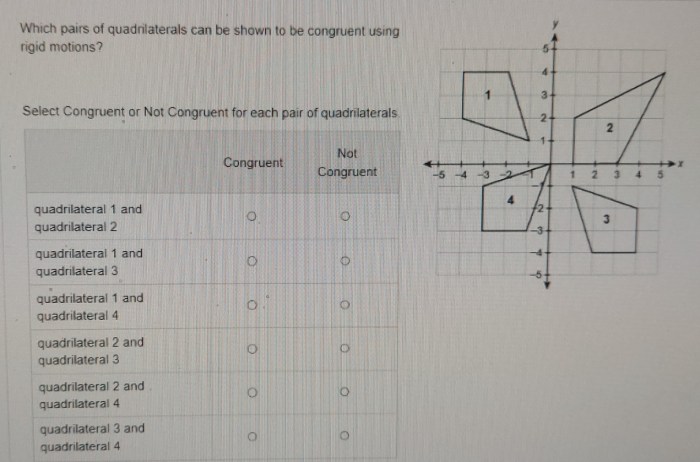
- Use transformations (rotations, translations, reflections) to align the shapes and check for congruence.
Transformations preserve the geometric properties of shapes, so if two shapes can be transformed into each other without changing their dimensions or angles, they are congruent.
Analyzing Given Shapes
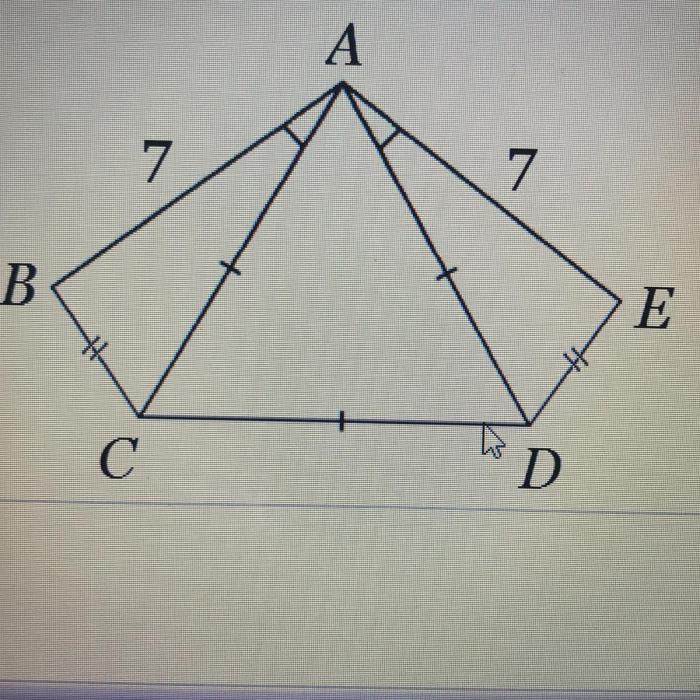
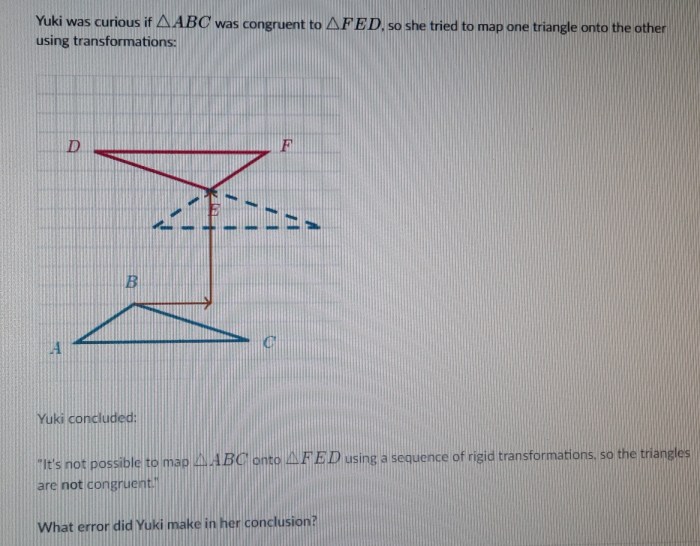
Consider the given shapes abc and aed:
Shape abc:
- Side lengths: ab = 5 cm, bc = 3 cm, ac = 4 cm
- Angles: ∠abc = 90°, ∠acb = 60°, ∠bac = 30°
Shape aed:
- Side lengths: ae = 5 cm, ed = 3 cm, ad = 4 cm
- Angles: ∠aed = 90°, ∠ade = 60°, ∠dae = 30°
Justifying Congruence or Non-Congruence
Based on the analysis, shapes abc and aed have equal side lengths (SSS) and equal angles (ASA). Therefore, by the ASA congruence condition, we can conclude that abc is congruent to aed.
Common Queries
What is the significance of congruence in geometry?
Congruence is a fundamental concept in geometry that establishes the equality of shapes and their corresponding parts. It plays a crucial role in understanding geometric relationships, solving complex problems, and designing structures.
How can we determine whether two shapes are congruent?
Determining congruence involves analyzing the shapes’ properties, such as side lengths, angles, and geometric relationships. Transformations like rotations, translations, and reflections can also be used to prove congruence.
What are the limitations of determining congruence?
Limitations may arise due to incomplete information or the presence of irregular shapes. Assumptions and approximations may be necessary in such cases, and the level of accuracy depends on the available data.